—————————— read pre-print on research gate (new window) ——————————

• θ_si can be measured as the polarization angle of quantum entangled X-rays at the degenerate frequency of a maximal Bell state. As an angle θ_si=3.26239 rad ± 2 μrad; as a momentum θ_si=3.26239030392(48) kg m s^-1 and with respect to the Target Frame, θ_si has no units. The relation of angle and mass is mathematically demonstrated, as well, by No-Ping Chen, et. al.
• c is the speed of light which may also be written as c=n_Ll_f/n_Tt_f=299,792,458 m/s such that n_L=n_T=1 is physically significant.

• Q_L is the fractional portion of a count of l_f when engaging in a more precise calculation.
• n_Lr describes the count of l_f representative of the position of an observable with respect to the frame of a center of mass.
• S is the unknown constant when resolving a description of gravity, latter replaced with θ_si.
• r is the distance between an observer and a target.
• G is the gravitational constant, 6.6740779428(56) 10^-11m³kg^-1s^-2 such that its value considers the effects of length contraction associated with discrete measure at the upper count limit. Italicized G identifies a measure not at the limit (e.g., G=6.6738448362(53) 10^-11m³kg^-1s^-2 at the blackbody demarcation).

When classical expressions are written in terms of physically significant units of measure and counts of those measures, an expression and value for the gravitational constant can be resolved with quantum correspondence to measure. There are two prerequisite inputs; they are c and θ_si where the latter describes half of the Planck momentum as well as the radial rate of universal expansion.

A Measurement Quantization (MQ) description of the gravitational constant is resolved as a difference between two frames, the discrete Internal Frame of the universe and the non-discrete System Frame of the universe. We describe gravitational curvature as the loss of the non-discrete count Q_L of l_f with each increment of elapsed time t_f.

To this we add a an additional constant. Describing momentum with respect to the Internal Frame, θ_si=3.26239030392(48) matches in magnitude the angular measure for the signal and idler of the polarization field with respect to the plain of X-rays when at their degenerate frequency. These measurements were first modeled and carried about by Shwartz and Harris and published in their 2011 paper entitled, Polarization Entangled Photons at X-Ray Energies.

Applications of MQ offer us the ability to resolve descriptions of phenomena not just quantum in precision, but also cosmological. For instance, one may replace a description of fundamental mass m_f with terms defined with respect to the universe^{(1,Eq. 88)}. As demonstrated below, a description of fundamental mass then becomes a description of the diameter and age of the universe.

Importantly, scaling between local and cosmological frames is physically supported both by gedankin experiments and comparison of measurements of phenomena that are understood to arise during different epochs. Paradoxes include descriptions of a speed of light that differ as a function of measurement scope (i.e., quantum, macroscopic and cosmological). Using MQ, we recognize that changes in the speed of light would result in a corresponding change in the ground state orbital of atoms. Looking at the spectral patterns of distant light from the earliest of stars supports the claim that the speed of light has not varied with elapsed time.

A second support regards descriptions of properties of early universe phenomena compared to their measure in the present epoch. For this we offer several descriptions, a majority describing the Cosmic Microwave Background (CMB). We can resolve a no parameter calculation of its age, quantity and present-day density and temperature. Correspondence between MQ calculations and measurement all align digit-for-digit and within the margin of uncertainty associated with each measure.

In short, we find support for scaling of the fundamental expression across the entire measurement domain.

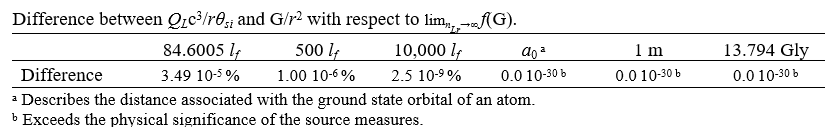
Let us now turn our attention to those properties associated with gravitational curvature. While we have used the equality symbol in the initial expressions to describe gravitational curvature^{(1,Eq. 8)}, said descriptions carry a prerequisite understanding of the conditions associated with their measure. The reason for this relates to the length contraction associated with discrete measure.

We find the effects of length contraction physically relevant in all descriptions incorporating the measures of G and ħ^{(4,Sec. III.E)}. Specifically, the value of G is a function of the distance associated with a phenomenon. In that G is typically measured macroscopically, for instance, where the measure of G is resolved at a distance of one meter, then the effects described by the Informativity differential are many orders of magnitude less than the precision afforded. The same would be true where we measured the reduced Planck constant as a function of a quantum distance (i.e., as is the case when measuring blackbody radiation). Combining these two measures considers the effects of length contraction associated with a discrete internal frame as a function of two frames of reference. This results in a disagreement in the proposed equality. For instance, using Planck's unit expressions to solve for the value of G will produce different results.

We can solve the problem by resolving a value for G and ħ at a given distance, both as a function of an electromagnetic phenomenon (a quantum distance) or both with respect to a macroscopic distance. Typically we solve measures at their upper count limit. When a term represents a measure at the upper count limit, we do not italicize the term.

A second observation is realized when G is described in terms of the fundamental measures^{(4,Eqs. 67-79)}. We discover the expression for G has two components. First, there is the length to time ratio (l_f/t_f), which we refer to as the length frequency. Second, there is the mass to time frequency, which we refer to as the mass frequency (m_f/t_f). While such descriptions are sometimes presented in terms of fundamental measures, it is understood that we are referring to the corresponding count terms (i.e., the count frequency). As such, the gravitational constant is length frequency cubed - once for each spatial dimension - divided by the mass frequency.

The expression describes length, mass and their relation to time. The correlation between mass and time is one dimensional. But, the correlation between length and time is three dimensional, representative of each of the three physical dimensions that describe space^{(2,Eq. 102)}. This is often a lost feature as physicists will typically reduce the expression to remove one iteration of t_f from the result.

We will lastly note, there are mathematical arguments that support greater dimensions in a description of space. MQ offers new tools to physically validate such claims. Where the Informativity differential is a function of the Pythagorean theorem - dimensions two or greater - it follows that any mathematical model employing dimensions two or greater with respect to any measure, must also account for the contraction of that measure for that dimension.