—————————— read pre-print on research gate (new window) ——————————

There are no inputs needed to resolve this expression.

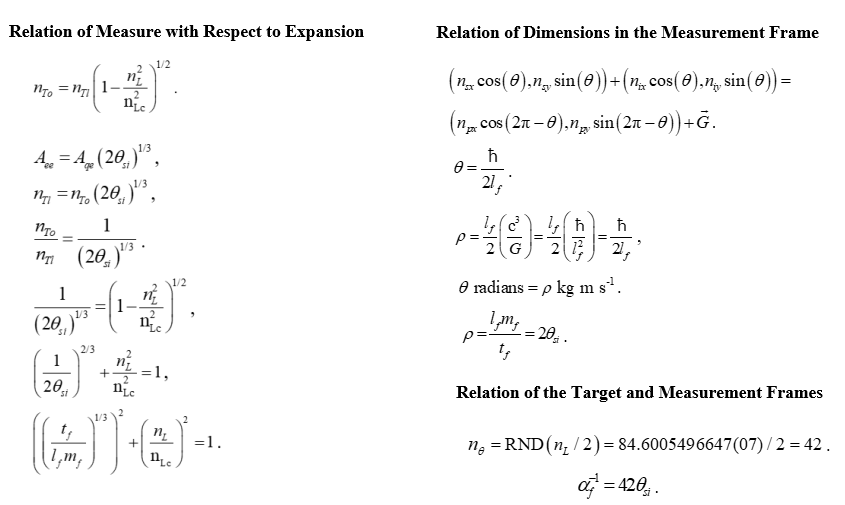
• l_f, m_f and t_f are the fundamental measures, more precise expressions for Planck’s units – length, mass, and time – that consider the effects of length contraction associated with discrete measure.
• n_L, n_M and n_T are physically significant discrete counts of l_f, m_f and t_f respectively.
• n_Lc describes the count of l_f representative of a change in position of light measured with respect to the observer’s frame of reference.

When using the unity expression to correlate the fundamental measures to universal expansion at t₀, an expression is resolved consisting of three terms, a sum of two squared ratios equal to 1. The expression is known as the Pythagorean Theorem.  Each squared term corresponds to a leg of a right-angle triangle. Importantly, we can present the expression such that Side a is the rate of expansion in three dimensions (i.e. the cube root of three) defined with respect to the universe (i.e. H_U=t_f /l_fm_f). The right term describes that expansion as a count change n_M (i.e. motion) with respect to the count limit n_Lc. The rate of expansion (n_L/n_Lc)² also takes the form of Einstein's speed parameter. In the case of the universe we then have a count ratio of increasing system length divided by the count change associated with the length frequency bound n_Lc).

Count bound ratios are important in Measurement Quantization (MQ). They arise regularly in physical descriptions and are essential to understanding phenomena such as discrete gravity, the expansion of the universe and galactic rotation. In principle, they are simplistic, for instance, the speed-of-light c=n_Ll_f/n_Tt_f. We call this the length frequency. The expression describes the upper count bound corresponding to a change in fundamental units of length per count of fundamental time. Historically, the physical significance of these unit measures has not been physically correlated to measure. But, with MQ their physical significance is resolved.

The remaining dimensional bounds are mass divided by time n_M/n_T which we refer to as the mass frequency and n_L/n_M which we refer to as the length-to-mass frequency. While these are each physically significant, there are other bounds that play an important role in nature. For one, the gravitational constant is the cube of length frequency divided by the mass frequency, described as a function of the corresponding reference measures G=(l_f/t_f)³/(m_f/t_f).

Most importantly, the expression constrains and defines measure.  That is, the three fundamental measures are now understood as a function of counts of those measures. Elapsed time describes the expansion and the length count bound n_L constrains the measures. As noted before, the rate of expansion is constant. Any variation in the rate of expansion can have an impact on the relative values of the fundamental measures, an emergent feature of the internal frame that underlies the values of the physical constants.