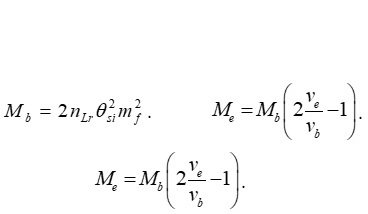—————————— read pre-print on research gate (new window) ——————————

• θ_si can be measured as the polarization angle of quantum entangled X-rays at the degenerate frequency of a maximal Bell state. As an angle θ_si=3.26239 rad ± 2 μrad; as a momentum θ_si=3.26239030392(48) kg m s^-1 and with respect to the Target Frame, θ_si has no units. The relation of angle and mass is mathematically demonstrated, as well, by No-Ping Chen, et. al.
• l_f, m_f and t_f are the fundamental measures, more precise expressions for Planck’s units – length, mass, and time – that consider the effects of length contraction associated with discrete measure.
• n_Lr describes the count of l_f representative of a change in position of an observable measured with respect to the observer’s frame of reference.
• v_m is the modelled velocity best reflecting the measurement data.
• v_e is the escape velocity associated with a gravitational mass.
• v_e is the effective velocity, a function of the effective mass – that count of m_f constrained by the Planck frequency – and reflecting the mass profile of a system.
• R is the distance between a center of mass and an inertial frame.

• v_b is the bound velocity that corresponds to the bound mass constrained by the Planck frequency.
• n_Lr describes the count of l_f representative of the position of an observable with respect to the frame of a center of mass.
• n_Lc describes the count of l_f representative of a change in position of light measured with respect to the observer’s frame of reference.
• H is the value of H0 m s-1Mpc-1 when resolved with respect to universal expansion (i.e., the Target Frame).
• H_U is the rate of universal expansion with respect to the diameter of the universe (per D_U). This differs from stellar expansion (i.e., Hubble’s description).
• c is the speed of light which may also be written as c=n_Ll_f/n_Tt_f=299,792,458 m/s such that n_L=n_T=1 is physically significant.
• M_tot is the total mass of the universe, including all forms of energy.
• M_acr is the rate of mass accretion in the universe.
• M_e is the effective mass which represents the gravitational mass measurable by an observer.
• M_b is the bound mass which represents the upper count bound of m_f that can be measured per t_f in a homogenous mass distribution.

While the mystery surrounding dark matter has been challenging to unravel, perhaps as puzzling is why star velocities are consistently much faster than expected across a galactic disk, yet roughly the same across the outer 2/3rds of a disk. One might conjecture there are two effects at work. We will describe both and identify their intersection.

In this article we specifically address what we call the quantization crossover. This is defined as that distance from a galactic core where the total mass of a system exceeds the measureable mass. Using the Measurement Quantization (MQ) approach to classical description, we resolve this distance at the Planck frequency bound, the upper count bound of fundamental units of mass m_f that can be measured per increment of fundamental time t_f.

Without going into too much detail regarding physical support for MQ, we refer the reader to several articles, noting here that MQ recognizes that the notion of measure is a count phenomenon with respect to length, mass and time references as resolved with respect to the Internal Frame of the universe. Likewise, it is shown that because the universe has no external reference, the System Frame of the universe must be non-discrete. And when considering the difference between these frames, we resolve values and expressions for the physical constants and the laws of nature.

Importantly, discrete measure where there are two or more dimensions associated with a measure (i.e., length) carries with it a contraction effect - the Informativity differential. This effect is not related to Einstein's relativity.

This effect can and has already been measured with respect to existing CODATA publications for the values of G and ħ. Over the last decade, there has been little agreement as to what these values should be. In the paper entitled, Measurement Quantization, we use our understanding of this effect to calculate G and ħ, thus identifying the conditions of each experiment used for the 2010, 2014, and 2018 publications.

We now turn our attention to count bounds, for instance, the count relation between fundamental length and time, often described with respect to the speed of light. We call this the length frequency bound. It describes the upper count bound of length measures per count of time measures. In that the fundamental measures are references and therein do not have smaller physically significant features such as curvature, we separate the counts from the measures. With this nomenclature, we recognize and can demonstrate that it is the counts that vary.

As such, we also recognize a mass frequency bound, that is the upper count bound n_M of mass measures with respect to a count n_T of time measures. And finally there is the length-to-mass count bound n_L/n_T.

With this foundation, we carry out calculations to resolve the bound velocity and then the associated bound mass for a system. This is resolved with respect to the Internal Frame of the observer and must then be transformed against the System Frame, by multiplying by θ_si. Thereafter, we contrast the relation with the effective velocity and corresponding effective mass and reduce.

The expression is complicated in that data regarding the mass distribution of a galaxy is difficult to gather. Most often, source data comes in the form of velocity measurements, such as that by McGaugh's mass model data representing the first 85,000 lightyears of the Milky Way. This data is then modelled using MOND to describe what is observed.

We refer the reader to the linked article on dark matter for details. As regards this calculation, our focus is identifying the quantization crossover, the point where the modelled mass increases above the bound mass. We have identified this distance at 9.072419 10³ light-years. This is the radial distance at which star velocities flat line. Thereafter all velocities are roughly equal for the remainder of stars across the disk.

We emphasize, not all galaxies will demonstrate flat lining across the entire disk. This behavior will only appear so long as the effective mass (red curve) exceeds the bound mass (purpose line). Even with respect to the Milky Way, this behvior disappears near the edge of the disk.